a uniform piece of sheet metal is shaped There are 2 steps to solve this one. Let's name the three unshaded regions as 1, 2, 3. Let A be the total area of the square. A 1, A 2 and A 3 are the area of. A uniform piece of sheet metal . $21.99
0 · Solved A uniform piece of sheet metal is shaped as shown in
1 · SOLVED: A uniform piece of sheet metal is shaped as shown in
2 · Q48P A uniform piece of sheet metal i [FREE SOLUTION]
3 · Center mass of uniform sheet of steel
4 · Answered: A uniform piece of sheet metal is
5 · A uniform piece of sheetmetal is shaped as shown in
6 · A uniform piece of sheet metal is shaped as shown in the
7 · A uniform piece of sheet metal is shaped as shown in
Wholesale aluminum cnc milling parts manufacturer provides custom service as well. Contact us for price of cnc milling aluminum parts. One-stop solution for Sheet Metal Fabrication & CNC Machining - Bergek CNC
Solved A uniform piece of sheet metal is shaped as shown in
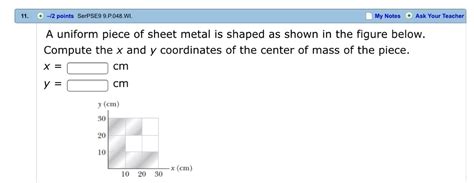
A uniform piece of sheet metal is shaped as shown in Figure P9.48. Compute the x and y coordinates of the center of mass of the piece.There are 2 steps to solve this one. Let's name the three unshaded regions as 1, 2, 3. Let A be the total area of the square. A 1, A 2 and A 3 are the area of. A uniform piece of sheet metal .A uniform sheet each of thickness 10 units is cut into the shape as shown. Compute then x and y-coordinates of the centre of mass of the piece from point A.A uniform piece of sheet metal is shaped as shown in the figure below. Compute the x and y coordinates of the center of mass of the piece.x = cmy = cmA graph shows a piece .
Uniform piece of sheet metal shaped as shown in the figure below. Compute the x and y coordinates of the center of mass of the piece. Mass of the piece: ______ (cm^2)We can do this by using the formula: xcm = (Σmixi) / Σmi where m is the mass of each small element of the shape, xi is the x-coordinate of that element, and Σmi is the total mass of the shape. Since the sheet metal is uniform, we can assume . First, we need to divide the shape into simpler shapes. We can divide it into a rectangle and a triangle. Next, we need to find the area of each shape. Let's call the width of .
Solution for A uniform piece of sheet metal is shaped as shown in the figure below. Compute the x and y coordinates of the center of mass of the piece. X = cm y. Center mass of a uniform sheet of steel can be calculated by finding the average of the x and y coordinates of all the individual mass elements that make up the sheet. This can .Solution for A uniform piece of sheet metal is shaped as shown in the figure below. Compute the x and y coordinates of the center of mass of the piece. X= cm y.A uniform piece of sheet metal is shaped as shown in Figure P9.48. Compute the x and y coordinates of the center of mass of the piece.
There are 2 steps to solve this one. Let's name the three unshaded regions as 1, 2, 3. Let A be the total area of the square. A 1, A 2 and A 3 are the area of. A uniform piece of sheet metal is shaped as shown in the figure below. Compute the x and y coordinates of the center of mass of the piece. x = cm y = cm y (cm) 30 20 10 -x (cm) 10 20 30.A uniform sheet each of thickness 10 units is cut into the shape as shown. Compute then x and y-coordinates of the centre of mass of the piece from point A.A uniform piece of sheet metal is shaped as shown in the figure below. Compute the x and y coordinates of the center of mass of the piece.x = cmy = cmA graph shows a piece of sheet metal placed on the first quadrant. The graph is shown to have nine cells, each with a width and height of 10 cm, with three cells on each row.
Uniform piece of sheet metal shaped as shown in the figure below. Compute the x and y coordinates of the center of mass of the piece. Mass of the piece: ______ (cm^2)We can do this by using the formula: xcm = (Σmixi) / Σmi where m is the mass of each small element of the shape, xi is the x-coordinate of that element, and Σmi is the total mass of the shape. Since the sheet metal is uniform, we can assume that the mass is evenly distributed throughout the shape.
First, we need to divide the shape into simpler shapes. We can divide it into a rectangle and a triangle. Next, we need to find the area of each shape. Let's call the width of the rectangle a, the height of the rectangle b, and the height of the triangle c. The area of the rectangle is A 1 = a b, and the area of the triangle is A 2 = 1 2 a c .
Solution for A uniform piece of sheet metal is shaped as shown in the figure below. Compute the x and y coordinates of the center of mass of the piece. X = cm y.

Center mass of a uniform sheet of steel can be calculated by finding the average of the x and y coordinates of all the individual mass elements that make up the sheet. This can be done using the formula x̄ = ∑mx/∑m and ȳ = ∑my/∑m, where m is the mass of each element and x and y are the coordinates of the element.
Solution for A uniform piece of sheet metal is shaped as shown in the figure below. Compute the x and y coordinates of the center of mass of the piece. X= cm y.A uniform piece of sheet metal is shaped as shown in Figure P9.48. Compute the x and y coordinates of the center of mass of the piece.There are 2 steps to solve this one. Let's name the three unshaded regions as 1, 2, 3. Let A be the total area of the square. A 1, A 2 and A 3 are the area of. A uniform piece of sheet metal is shaped as shown in the figure below. Compute the x and y coordinates of the center of mass of the piece. x = cm y = cm y (cm) 30 20 10 -x (cm) 10 20 30.A uniform sheet each of thickness 10 units is cut into the shape as shown. Compute then x and y-coordinates of the centre of mass of the piece from point A.
A uniform piece of sheet metal is shaped as shown in the figure below. Compute the x and y coordinates of the center of mass of the piece.x = cmy = cmA graph shows a piece of sheet metal placed on the first quadrant. The graph is shown to have nine cells, each with a width and height of 10 cm, with three cells on each row.
SOLVED: A uniform piece of sheet metal is shaped as shown in
Uniform piece of sheet metal shaped as shown in the figure below. Compute the x and y coordinates of the center of mass of the piece. Mass of the piece: ______ (cm^2)We can do this by using the formula: xcm = (Σmixi) / Σmi where m is the mass of each small element of the shape, xi is the x-coordinate of that element, and Σmi is the total mass of the shape. Since the sheet metal is uniform, we can assume that the mass is evenly distributed throughout the shape.
First, we need to divide the shape into simpler shapes. We can divide it into a rectangle and a triangle. Next, we need to find the area of each shape. Let's call the width of the rectangle a, the height of the rectangle b, and the height of the triangle c. The area of the rectangle is A 1 = a b, and the area of the triangle is A 2 = 1 2 a c .Solution for A uniform piece of sheet metal is shaped as shown in the figure below. Compute the x and y coordinates of the center of mass of the piece. X = cm y. Center mass of a uniform sheet of steel can be calculated by finding the average of the x and y coordinates of all the individual mass elements that make up the sheet. This can be done using the formula x̄ = ∑mx/∑m and ȳ = ∑my/∑m, where m is the mass of each element and x and y are the coordinates of the element.
![Q48P A uniform piece of sheet metal i [FREE SOLUTION]](/upluds/images/Q48P A uniform piece of sheet metal i [FREE SOLUTION] .jpg)
metal box not safe for eating
$119.54
a uniform piece of sheet metal is shaped|Q48P A uniform piece of sheet metal i [FREE SOLUTION]